Published online by Cambridge University Press: 26 November 2024
We show that a compact complex manifold X has no non-trivial nef  $(1,1)$-classes if there is a non-biholomorphic bimeromorphic map
$(1,1)$-classes if there is a non-biholomorphic bimeromorphic map 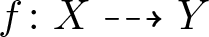 $f\colon X\dashrightarrow Y$, which is an isomorphism in codimension 1 to a compact Kähler manifold Y with
$f\colon X\dashrightarrow Y$, which is an isomorphism in codimension 1 to a compact Kähler manifold Y with 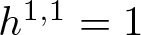 $h^{1,1}=1$. In particular, there exist infinitely many isomorphic classes of smooth compact Moishezon threefolds with no nef and big
$h^{1,1}=1$. In particular, there exist infinitely many isomorphic classes of smooth compact Moishezon threefolds with no nef and big 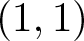 $(1,1)$-classes. This contradicts a recent paper (Strongly Jordan property and free actions of non-abelian free groups, Proc. Edinb. Math. Soc., 65(3) (2022), 736–746).
$(1,1)$-classes. This contradicts a recent paper (Strongly Jordan property and free actions of non-abelian free groups, Proc. Edinb. Math. Soc., 65(3) (2022), 736–746).