Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-28T08:57:48.220Z Has data issue: false hasContentIssue false
  • English
  • Français

Thin sets in

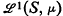
Published online by Cambridge University Press:  20 January 2009

I. Tweddle
I. Tweddle
Affiliation:
University of Stirling, Stirling
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In (4) J. F. C. Kingman and A. P. Robertson introduced the idea of thin sets in certain ℒ1 spaces. Thin sets are extreme cases of sets which are not total, and so the problem naturally arises of partitioning a measure space relative to a given set of integrable functions in such a way that on each element of the partition, the set of functions is either thin or total in a sense which is made precise below. In the present note, such partitions are obtained in §2 for finite or totally σ-finite measure spaces. In §3 the basic ideas are reformulated in terms of Radon measures on locally compact spaces, leading to an extension of the results of §2 in this context.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 17 , Issue 4 , December 1971 , pp. 311 - 316
Copyright
Copyright © Edinburgh Mathematical Society 1971

References

REFERENCES

(1) Bourbaki, N., Espaces vectoriels topologiques (Hermann, Paris 1966, 1967).Google Scholar
(2) Bourbaki, N., Intégration (Hermann, Paris 1965, 1967).Google Scholar
(3) Dieudonné, J., Foundations of Modern Analysis (Academic Press, New York (1960).Google Scholar
(4) Kingman, J. F. C. and Robertson, A. P., On a theorem of Lyapunov, J. London Math. Soc. 43 (1968), 347–351.CrossRefGoogle Scholar