Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-25T00:30:21.993Z Has data issue: false hasContentIssue false
  • English
  • Français

Useful Theorems on Commutative Non-associative Algebras

Published online by Cambridge University Press:  20 January 2009

Nora Losey
Nora Losey
Affiliation:
University of Manitoba
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Recently J. M. Osborn has investigated the structure of a simple commutative non-associative algebra with unity element satisfying a polynomial identity, (4), (5) and (6). From his work it seems likely that if such an algebra is of degree three or more it is necessarily power-associative. In (4) he establishes a hierarchy of identities with the property that each identity is satisfied by an algebra satisfying no preceding identity. Following (5), (6),the next identity to consider is

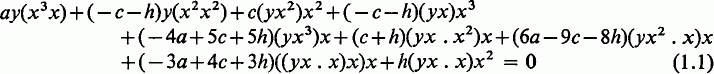
where a, c and h are elements of the ground field.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 15 , Issue 3 , June 1967 , pp. 203 - 208
Copyright
Copyright © Edinburgh Mathematical Society 1967

References

REFERENCES

(1) Albert, A. A., A structure theory for Jordan algebras, Ann. Math. 48 (1947), 546–567.CrossRefGoogle Scholar
(2) Albert, A. A., On the power-associativity of rings, Summa Brasiliensis Mathematicae, 2 (1948), 21–23.Google Scholar
(3) Albert, A. A., A theory of power-associative commutative algebras, Trans. Amer. Math. Soc. 69 (1950), 503–527.CrossRefGoogle Scholar
(4) Osborn, J. M., Identities on non-associative algebras, Canadian J. Math. 17 (1965), 78–92.CrossRefGoogle Scholar
(5) Osborn, J. M., An identity of degree four, Proc. Amer. Math. Soc. 16 (1965), 1114–1119.CrossRefGoogle Scholar
(6) Osborn, J. M., A generalization of power-associativity, to appear in Pacific J. Math.Google Scholar
(7) Osborn, J. M., On commutative non-associative algebras, J. Algebra, 2 (1965), 48–79.CrossRefGoogle Scholar