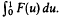Since the work of R. A. Fisher [2] and Kolmogorov, Petrovskij and Piskunov [7] (see [6] for further references) the problem of travelling fronts in reaction–diffusion equations has been extensively studied. For the equation

with F(0) = F(1) = 0 a travelling front is a solution

where the function of one variable φ is decreasing and satisfies φ(−∞) = 1, φ(+∞) = 0. The function φ describes the shape of the front and the constant c is the speed of propagation. There are two main types of the problem. In the all-positive case, where the function F satisfies

there is a half-line [c0, ∞), c0>0, of speeds. For each c∈[c0, ∞) there is, up to translation, a unique travelling front. Fronts for different c can be distinguished by the rate of decay towards +∞. In the threshold case, where F has the property, for some λ∈(0, 1),

there is a unique speed c0 with a travelling front, which is unique up to translation. In this case the sign of c0 is determined by the sign of the integral