Article contents
Some results for semi-stable radial solutions of k-Hessian equations with weight on ℝn
Published online by Cambridge University Press: 15 November 2022
Abstract
We devote this paper to study semi-stable nonconstant radial solutions of $S_k(D^2u)=w(\left \vert x \right \vert )g(u)$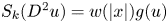 on the Euclidean space $\mathbb {R}^n$
on the Euclidean space $\mathbb {R}^n$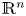 . We establish pointwise estimates and necessary conditions for the existence of such solutions (not necessarily bounded) for this equation. For bounded solutions we estimate their asymptotic behaviour at infinity. All the estimates are given in terms of the spatial dimension $n$
. We establish pointwise estimates and necessary conditions for the existence of such solutions (not necessarily bounded) for this equation. For bounded solutions we estimate their asymptotic behaviour at infinity. All the estimates are given in terms of the spatial dimension $n$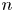 , the values of $k$
, the values of $k$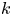 and the behaviour at infinity of the growth rate function of $w$
and the behaviour at infinity of the growth rate function of $w$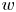 .
.
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 153 , Issue 5 , October 2023 , pp. 1751 - 1776
- Copyright
- Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh
References
- 1
- Cited by



