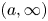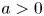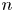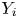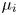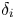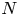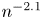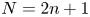Research Article
Depths in hooking networks
-
- Published online by Cambridge University Press:
- 11 May 2021, pp. 941-949
-
- Article
- Export citation
Stochastic comparison of parallel systems with Pareto components
- Part of:
-
- Published online by Cambridge University Press:
- 20 May 2021, pp. 950-962
-
- Article
- Export citation
Designing a Bonus-Malus system reflecting the claim size under the dependent frequency–severity model
-
- Published online by Cambridge University Press:
- 07 June 2021, pp. 963-987
-
- Article
- Export citation
Team's seasonal win probabilities
-
- Published online by Cambridge University Press:
- 02 July 2021, pp. 988-998
-
- Article
- Export citation
Temporal concatenation for Markov decision processes
-
- Published online by Cambridge University Press:
- 13 July 2021, pp. 999-1026
-
- Article
- Export citation
Equivalency in joint signatures for binary/multi-state systems of different sizes
-
- Published online by Cambridge University Press:
- 14 July 2021, pp. 1027-1054
-
- Article
- Export citation
Stochastic properties of generalized finite α-mixtures
-
- Published online by Cambridge University Press:
- 15 July 2021, pp. 1055-1079
-
- Article
-
- You have access
- HTML
- Export citation
On history-dependent mixed shock models
-
- Published online by Cambridge University Press:
- 06 August 2021, pp. 1080-1097
-
- Article
- Export citation
On the distribution of winners’ scores in a round-robin tournament
- Part of:
-
- Published online by Cambridge University Press:
- 06 August 2021, pp. 1098-1102
-
- Article
- Export citation
A closed-form pricing formula for catastrophe equity options
-
- Published online by Cambridge University Press:
- 15 July 2021, pp. 1103-1115
-
- Article
- Export citation
Ordering results for smallest claim amounts from two portfolios of risks with dependent heterogeneous exponentiated location-scale claims
- Part of:
-
- Published online by Cambridge University Press:
- 26 July 2021, pp. 1116-1137
-
- Article
- Export citation
A lattice approach for option pricing under a regime-switching GARCH-jump model
-
- Published online by Cambridge University Press:
- 29 July 2021, pp. 1138-1170
-
- Article
- Export citation
Concentrated matrix exponential distributions with real eigenvalues
-
- Published online by Cambridge University Press:
- 26 August 2021, pp. 1171-1187
-
- Article
- Export citation
Assortativity and bidegree distributions on Bernoulli random graph superpositions
-
- Published online by Cambridge University Press:
- 19 August 2021, pp. 1188-1213
-
- Article
- Export citation
A closed-form approximation formula for pricing European options under a three-factor model
-
- Published online by Cambridge University Press:
- 18 August 2021, pp. 1214-1240
-
- Article
- Export citation
Game theoretic modeling of economic denial of sustainability (EDoS) attack in cloud computing
-
- Published online by Cambridge University Press:
- 18 August 2021, pp. 1241-1265
-
- Article
- Export citation
A regret lower bound for assortment optimization under the capacitated MNL model with arbitrary revenue parameters
-
- Published online by Cambridge University Press:
- 01 September 2021, pp. 1266-1274
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Orderings of component level versus system level at active redundancies for coherent systems with dependent components
-
- Published online by Cambridge University Press:
- 10 September 2021, pp. 1275-1297
-
- Article
- Export citation
Front Cover (OFC, IFC) and matter
PES volume 36 issue 4 Cover and Front matter
-
- Published online by Cambridge University Press:
- 04 November 2022, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (IBC, OBC) and matter
PES volume 36 issue 4 Cover and Back matter
-
- Published online by Cambridge University Press:
- 04 November 2022, pp. b1-b2
-
- Article
-
- You have access
- Export citation