Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-22T18:33:32.706Z Has data issue: false hasContentIssue false
  • English
  • Français

Bounded Hankel Products on the Bergman Space of the Polydisk

Part of: Special classes of linear operators

Published online by Cambridge University Press:  20 November 2018

Yufeng Lu  and
Shuxia Shang
Yufeng Lu
Affiliation:
Department of Applied Mathematics, Dalian University of Technology, Dalian 116024, China, lyfdlut1@yahoo.com.cnshangshux@163.com
Shuxia Shang
Affiliation:
Department of Applied Mathematics, Dalian University of Technology, Dalian 116024, China, lyfdlut1@yahoo.com.cnshangshux@163.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider the problem of determining for which square integrable functions 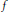$f$ and $g$ on the polydisk the densely defined Hankel product 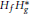${{H}_{f}}\,H_{g}^{*}$ is bounded on the Bergman space of the polydisk. Furthermore, we obtain similar results for the mixed Haplitz products 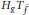${{H}_{g}}\,{{T}_{{\bar{f}}}}$ and 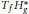${{T}_{f}}\,H_{g}^{*}$, where 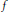$f$ and $g$ are square integrable on the polydisk and 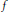$f$ is analytic.

Keywords

Toeplitz operatorHankel operatorHaplitz productsBergman spacepolydisk

MSC classification

Primary: 47B35: Toeplitz operators, Hankel operators, Wiener-Hopf operators
Secondary: 47B47: Commutators, derivations, elementary operators, etc.
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 61 , Issue 1 , 01 February 2009 , pp. 190 - 204
Copyright
Copyright © Canadian Mathematical Society 2009

References

[1] Rudin, W., Real and complex analysis. McGraw-Hill Book Co., New York, 1966.Google Scholar
[2] Stroethoff, K. and Zheng, D., Products of Hankel and Toeplitz operators on the Bergman space. J. Funct. Anal. 169 (1999), no. 1, 289–313.Google Scholar
[3] Stroethoff, K. and Zheng, D., Bounded Toeplitz products on the Bergman space of the polydisk. J. Math. Anal. Appl. 278 (2003), no. 1, 125–135.Google Scholar
[4] Stroethoff, K. and Zheng, D., Algebraic and spectral properties of dual Toeplitz operators. Trans. Amer. Math. Soc. 354 (2002), no. 6, 2495–2520.Google Scholar
[5] Stroethoff, K. and Zheng, D., Toeplitz and Hankel operators on Bergman spaces. Trans. Amer. Math. Soc. 329 (1992), no. 2, 773–794.Google Scholar
[6] Lu, Y., Commuting dual Toeplitz operators with pluriharmonic symbols. J. Math. Anal. Appl. 302 (2005), no. 1, 149–156.Google Scholar
[7] Zhu, K. H., Operator theory in function spaces. Monographs and Textbooks in Pure and Applied Mathematics 139, Marcel Dekker, New York, 1990.Google Scholar