Contents
Research Article
On the Fredholm integral equation associated with pairs of dual integral equations
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 185-194
-
- Article
-
- You have access
- Export citation
On radicals of infinite matrix rings
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 195-203
-
- Article
-
- You have access
- Export citation
Continuous functions of bounded nth variation
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 205-214
-
- Article
-
- You have access
- Export citation
Semi-simple locally compact monothetic semi-algebras
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 215-219
-
- Article
-
- You have access
- Export citation
Arithmetical identities and Hecke's functional equation
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 221-226
-
- Article
-
- You have access
- Export citation
On Riesz operators
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 227-232
-
- Article
-
- You have access
- Export citation
Bounds for permanents of non-negative matrices1
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 233-237
-
- Article
-
- You have access
- Export citation
On distributively generated near-rings1
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 239-243
-
- Article
-
- You have access
- Export citation
On Kadison's condition for extreme points of the unit ball in a B*-algebra
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 245-250
-
- Article
-
- You have access
- Export citation
An exclusion theorem for tri-diagonal matrices
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 251-253
-
- Article
-
- You have access
- Export citation
Fundamental solutions and surface distributions
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 255-257
-
- Article
-
- You have access
- Export citation
On a Boolean algebra of projections constructed by Dieudonné
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 259-262
-
- Article
-
- You have access
- Export citation
On partitions of N points
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 263-264
-
- Article
-
- You have access
- Export citation
Book Reviews
V. I. Smirmov, A Course of Higher Mathematics, Vol. I: Elementary Calculus, 546 pp., 90s.; Vol. II: Advanced Calculus, 632 pp., 90s.; Vol. III, Part 1: Linear Algebra, 326 pp., 63s.; Vol. III, Part 2: Complex Variables and Special Functions, 702 pp., 110s.; Vol. IV: Integral Equations and Partial Differential Equations, 814 pp., 126s.
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 265-266
-
- Article
-
- You have access
- Export citation
M. Bruckheimer, N. W. Gowar, and R. E. Scraton, Mathematics for Technology: A New Approach (Chatto and Windus, London, 1968), xiv+558 pp., 48s.
-
- Published online by Cambridge University Press:
- 20 January 2009, p. 266
-
- Article
-
- You have access
- Export citation
F. Treves Locally Convex Spaces and Linear Partial Differential Equations (Springer-Verlag, Berlin, 1967), xii+121 pp., DM 36.
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 266-267
-
- Article
-
- You have access
- Export citation
E. T. Copson, Metric Spaces, Cambridge Tracts in Mathematics and Mathematical Physics No. 57 (Cambridge University Press, 1968), 30s.
-
- Published online by Cambridge University Press:
- 20 January 2009, p. 267
-
- Article
-
- You have access
- Export citation
Richard D. Schafer, An Introduction to Nonassociative Algebras (Academic Press Inc., New York and London, 1966), x+166 pp., 64s.
-
- Published online by Cambridge University Press:
- 20 January 2009, pp. 267-268
-
- Article
-
- You have access
- Export citation
Francis B. Hildebrand, Finite Difference Equations and Simulations (Prentice Hall, 1968), ix+338 pp., 119s. 6d.
-
- Published online by Cambridge University Press:
- 20 January 2009, p. 268
-
- Article
-
- You have access
- Export citation
Z. I. Borevich and I. R. Shafarevich, Number Theory (Academic Pres, New York and London, 1966), x+435 pp., 104s.
-
- Published online by Cambridge University Press:
- 20 January 2009, p. 269
-
- Article
-
- You have access
- Export citation

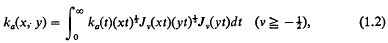
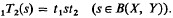
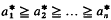 be the numbers
be the numbers 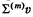 denote the sum of
denote the sum of 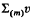 the sum of
the sum of 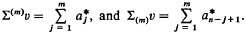
 of elements
of elements 

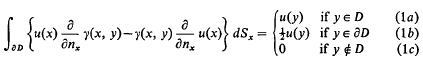
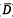

 denotes differentiation along the inward normal to ∂
denotes differentiation along the inward normal to ∂